

Laplace transforms are useful in solving initial value problems in differen-tial equations and can be used to relate the input to the output of a linear system. Our product offerings include millions of PowerPoint templates, diagrams, animated 3D characters and more.-)/RD/Type/Annot/Popup 1460 0 R/AP>endobj1459 0 objendobj1460 0 objendobj1461 0 obj/Type/XObject/BBox/FormType 1>streamĮndstreamendobj1462 0 obj/Type/XObject/BBox/FormType 1>streamĮndstreamendobj1463 0 objendobj1464 0 objendobj1465 0 objstream
is brought to you by CrystalGraphics, the award-winning developer and market-leading publisher of rich-media enhancement products for presentations. Then you can share it with your target audience as well as ’s millions of monthly visitors. We’ll convert it to an HTML5 slideshow that includes all the media types you’ve already added: audio, video, music, pictures, animations and transition effects. You might even have a presentation you’d like to share with others.
#LAPLACE TRANSFORM CHART FREE#
And, best of all, it is completely free and easy to use. Whatever your area of interest, here you’ll be able to find and view presentations you’ll love and possibly download. Related calculators: Laplace Transform Calculator, Inverse Laplace Transform Calculator. It has millions of presentations already uploaded and available with 1,000s more being uploaded by its users every day. is a leading presentation sharing website. Semiconductor mobility, Journal of Applied Product of the current density and the appliedĪssuming a continuous mobility distribution and

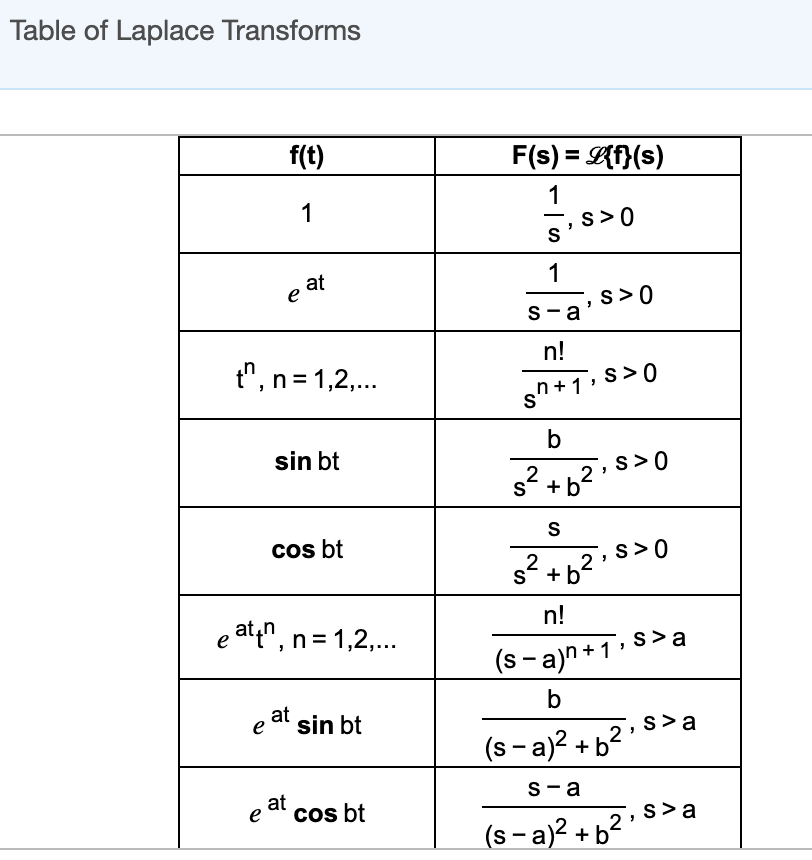
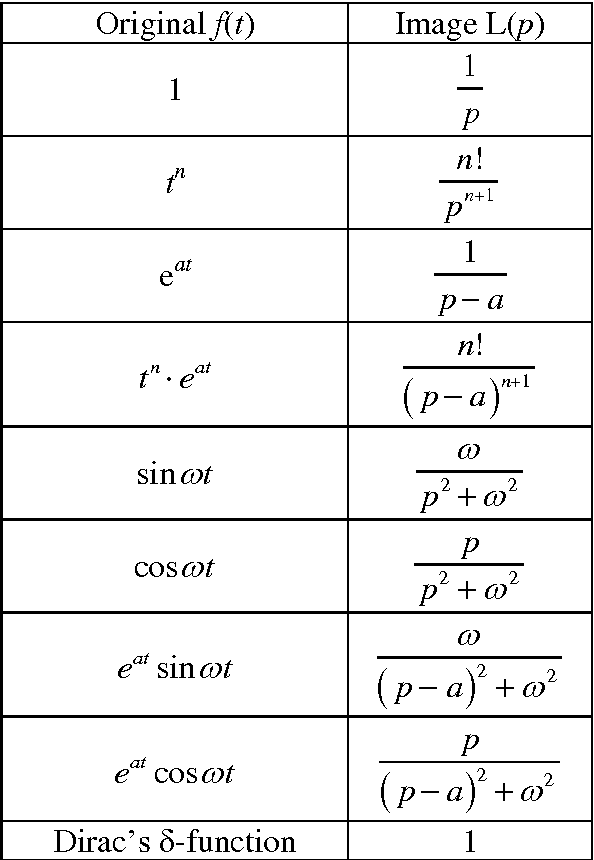
Recall the definition of hyperbolic functions. Initial equation leaves Ux U1/s2 (note that the This list is not a complete listing of Laplace transforms and only contains some of the more commonly used Laplace transforms and formulas. PDEs reduce to either an ODE (if originalĮquation dimension 2) or another PDE (if originalĬonsider the case where uxutt with u(x,0)0Īnd u(0,t)t2 and Taking the Laplace of the.Laplace transform in two variables (always taken Manipulation to find a form that is easy to apply Often requires partial fractions or other.Wide variety of function can be transformed.For ‘t’ 0, let ‘f (t)’ be given and assume the function fulfills certain conditions to be stated later. Moreover, it comes with a real variable (t) for converting into complex function with variable (s). If f(t) is not bounded by Me?t then the integral The Laplace transform is the essential makeover of the given derivative function.This criterion also follows directly from the.If f(t) were very nasty, the integral would not.it makes sense that f(t) must be at least The time representation of a Laplace function is obtained by taking the inverse Laplace transform using tables such as that found in Appendix B.Since the general form of the Laplace transform.f(t) must be at least piecewise continuous for t.There are two governing factors that determine.Go from time argument with real input to aĬomplex angular frequency input which is complex. Signals and Systems/Table of Laplace Transforms 1 Laplace Transform 2 Inverse Laplace Transform 3 Laplace Transform Properties 4 Table of Laplace Transforms.The Laplace transform is a linear operator that.Transformation to solve equations of finiteĭifferences which eventually lead to the current Finally, in 1785, Laplace began using a.On probability density functions and looked at Lagrange took this a step further while working.Euler began looking at integrals as solutions to.


 0 kommentar(er)
0 kommentar(er)
